Cive 302 solid mechanics lab report

⭳ 45 Download 📄 9 Pages / 2228 Words

Data:

X (in)	100lb at P1 (30 in)	100lb at P2 (60 in)	100lb at P1/P2
d1	5	-0.011	-0.0085	-0.0195
d2	25	-0.0455	-0.04	-0.085
d3	45	-0.054	-0.055	-0.1085
d4	65	-0.038	-0.046	-0.084
d5	85	-0.0075	-0.0095	-0.0175

Table 1: Experimental displacement readings

LC1	LC2	LC3
P1, lbs	100	0	100
P2, lbs	0	100	100
C1	-50000	-40000	-90000
E, ksi	10000
I, in^4	2.5
L, in	90
c, in	1.5

Table 2: Given values

Calculations:

x (in)	Moment LC1, lb-in	Moment LC2, lb-in	Moment LC3, lb-in	LC1 disp	LC2 disp	LC3 disp
0	0	0	0	0	0	0
0.5	33.3333	16.6667	50	-0.0010	-0.0008	-0.0018
1	66.6667	33.3333	100	-0.0020	-0.0016	-0.0036
1.5	100.0000	50.0000	150	-0.0030	-0.0024	-0.0054
2	133.3333	66.6667	200	-0.0040	-0.0032	-0.0072
2.5	166.6667	83.3333	250	-0.0050	-0.0040	-0.0090
3	200.0000	100.0000	300	-0.0060	-0.0048	-0.0108
3.5	233.3333	116.6667	350	-0.0070	-0.0056	-0.0126
4	266.6667	133.3333	400	-0.0080	-0.0064	-0.0144
4.5	300.0000	150.0000	450	-0.0090	-0.0072	-0.0161
5	333.3333	166.6667	500	-0.0099	-0.0080	-0.0179
5.5	366.6667	183.3333	550	-0.0109	-0.0088	-0.0197
6	400.0000	200.0000	600	-0.0119	-0.0096	-0.0215
6.5	433.3333	216.6667	650	-0.0129	-0.0103	-0.0232
7	466.6667	233.3333	700	-0.0138	-0.0111	-0.0250
7.5	500.0000	250.0000	750	-0.0148	-0.0119	-0.0267
8	533.3333	266.6667	800	-0.0158	-0.0127	-0.0285
8.5	566.6667	283.3333	850	-0.0167	-0.0135	-0.0302
9	600.0000	300.0000	900	-0.0177	-0.0142	-0.0319
9.5	633.3333	316.6667	950	-0.0186	-0.0150	-0.0336
10	666.6667	333.3333	1000	-0.0196	-0.0158	-0.0353
10.5	700.0000	350.0000	1050	-0.0205	-0.0165	-0.0370
11	733.3333	366.6667	1100	-0.0214	-0.0173	-0.0387
11.5	766.6667	383.3333	1150	-0.0223	-0.0181	-0.0404
12	800.0000	400.0000	1200	-0.0232	-0.0188	-0.0420
12.5	833.3333	416.6667	1250	-0.0241	-0.0196	-0.0437
13	866.6667	433.3333	1300	-0.0250	-0.0203	-0.0453
13.5	900.0000	450.0000	1350	-0.0259	-0.0211	-0.0470
14	933.3333	466.6667	1400	-0.0268	-0.0218	-0.0486
14.5	966.6667	483.3333	1450	-0.0276	-0.0225	-0.0502
15	1000.0000	500.0000	1500	-0.0285	-0.0233	-0.0518
15.5	1033.3333	516.6667	1550	-0.0293	-0.0240	-0.0533
16	1066.6667	533.3333	1600	-0.0302	-0.0247	-0.0549
16.5	1100.0000	550.0000	1650	-0.0310	-0.0254	-0.0564
17	1133.3333	566.6667	1700	-0.0318	-0.0261	-0.0579
17.5	1166.6667	583.3333	1750	-0.0326	-0.0268	-0.0594
18	1200.0000	600.0000	1800	-0.0334	-0.0275	-0.0609
18.5	1233.3333	616.6667	1850	-0.0342	-0.0282	-0.0624
19	1266.6667	633.3333	1900	-0.0350	-0.0289	-0.0638
19.5	1300.0000	650.0000	1950	-0.0357	-0.0296	-0.0653
20	1333.3333	666.6667	2000	-0.0364	-0.0302	-0.0667
20.5	1366.6667	683.3333	2050	-0.0372	-0.0309	-0.0681
21	1400.0000	700.0000	2100	-0.0379	-0.0315	-0.0694
21.5	1433.3333	716.6667	2150	-0.0386	-0.0322	-0.0708
22	1466.6667	733.3333	2200	-0.0393	-0.0328	-0.0721
22.5	1500.0000	750.0000	2250	-0.0399	-0.0335	-0.0734
23	1533.3333	766.6667	2300	-0.0406	-0.0341	-0.0747
23.5	1566.6667	783.3333	2350	-0.0412	-0.0347	-0.0759
24	1600.0000	800.0000	2400	-0.0419	-0.0353	-0.0772
24.5	1633.3333	816.6667	2450	-0.0425	-0.0359	-0.0784
25	1666.6667	833.3333	2500	-0.0431	-0.0365	-0.0796
25.5	1700.0000	850.0000	2550	-0.0436	-0.0371	-0.0807
26	1733.3333	866.6667	2600	-0.0442	-0.0377	-0.0819
26.5	1766.6667	883.3333	2650	-0.0447	-0.0383	-0.0830
27	1800.0000	900.0000	2700	-0.0453	-0.0388	-0.0841
27.5	1833.3333	916.6667	2750	-0.0458	-0.0394	-0.0851
28	1866.6667	933.3333	2800	-0.0462	-0.0399	-0.0862
28.5	1900.0000	950.0000	2850	-0.0467	-0.0405	-0.0872
29	1933.3333	966.6667	2900	-0.0472	-0.0410	-0.0881
29.5	1966.6667	983.3333	2950	-0.0476	-0.0415	-0.0891
30	2000.0000	1000.0000	3000	-0.0480	-0.0420	-0.0900
30.5	1983.3333	1016.6667	3000	-0.0484	-0.0425	-0.0909
31	1966.6667	1033.3333	3000	-0.0488	-0.0430	-0.0917
31.5	1950.0000	1050.0000	3000	-0.0491	-0.0435	-0.0926
32	1933.3333	1066.6667	3000	-0.0494	-0.0439	-0.0934
32.5	1916.6667	1083.3333	3000	-0.0498	-0.0444	-0.0941
33	1900.0000	1100.0000	3000	-0.0500	-0.0448	-0.0949
33.5	1883.3333	1116.6667	3000	-0.0503	-0.0452	-0.0956
34	1866.6667	1133.3333	3000	-0.0506	-0.0457	-0.0962
34.5	1850.0000	1150.0000	3000	-0.0508	-0.0461	-0.0969
35	1833.3333	1166.6667	3000	-0.0510	-0.0465	-0.0975
35.5	1816.6667	1183.3333	3000	-0.0512	-0.0469	-0.0981
36	1800.0000	1200.0000	3000	-0.0514	-0.0472	-0.0986
36.5	1783.3333	1216.6667	3000	-0.0516	-0.0476	-0.0992
37	1766.6667	1233.3333	3000	-0.0517	-0.0479	-0.0997
37.5	1750.0000	1250.0000	3000	-0.0518	-0.0483	-0.1001
38	1733.3333	1266.6667	3000	-0.0520	-0.0486	-0.1006
38.5	1716.6667	1283.3333	3000	-0.0520	-0.0489	-0.1010
39	1700.0000	1300.0000	3000	-0.0521	-0.0492	-0.1013
39.5	1683.3333	1316.6667	3000	-0.0522	-0.0495	-0.1017
40	1666.6667	1333.3333	3000	-0.0522	-0.0498	-0.1020
40.5	1650.0000	1350.0000	3000	-0.0522	-0.0500	-0.1023
41	1633.3333	1366.6667	3000	-0.0523	-0.0503	-0.1025
41.5	1616.6667	1383.3333	3000	-0.0522	-0.0505	-0.1028
42	1600.0000	1400.0000	3000	-0.0522	-0.0507	-0.1030
42.5	1583.3333	1416.6667	3000	-0.0522	-0.0509	-0.1031
43	1566.6667	1433.3333	3000	-0.0521	-0.0511	-0.1033
43.5	1550.0000	1450.0000	3000	-0.0521	-0.0513	-0.1034
44	1533.3333	1466.6667	3000	-0.0520	-0.0515	-0.1034
44.5	1516.6667	1483.3333	3000	-0.0519	-0.0516	-0.1035
45	1500.0000	1500.0000	3000	-0.0518	-0.0518	-0.1035
45.5	1483.3333	1516.6667	3000	-0.0516	-0.0519	-0.1035
46	1466.6667	1533.3333	3000	-0.0515	-0.0520	-0.1034
46.5	1450.0000	1550.0000	3000	-0.0513	-0.0521	-0.1034
47	1433.3333	1566.6667	3000	-0.0511	-0.0521	-0.1033
47.5	1416.6667	1583.3333	3000	-0.0509	-0.0522	-0.1031
48	1400.0000	1600.0000	3000	-0.0507	-0.0522	-0.1030
48.5	1383.3333	1616.6667	3000	-0.0505	-0.0522	-0.1028
49	1366.6667	1633.3333	3000	-0.0503	-0.0523	-0.1025
49.5	1350.0000	1650.0000	3000	-0.0500	-0.0522	-0.1023
50	1333.3333	1666.6667	3000	-0.0498	-0.0522	-0.1020
50.5	1316.6667	1683.3333	3000	-0.0495	-0.0522	-0.1017
51	1300.0000	1700.0000	3000	-0.0492	-0.0521	-0.1013
51.5	1283.3333	1716.6667	3000	-0.0489	-0.0520	-0.1010
52	1266.6667	1733.3333	3000	-0.0486	-0.0520	-0.1006
52.5	1250.0000	1750.0000	3000	-0.0483	-0.0518	-0.1001
53	1233.3333	1766.6667	3000	-0.0479	-0.0517	-0.0997
53.5	1216.6667	1783.3333	3000	-0.0476	-0.0516	-0.0992
54	1200.0000	1800.0000	3000	-0.0472	-0.0514	-0.0986
54.5	1183.3333	1816.6667	3000	-0.0469	-0.0512	-0.0981
55	1166.6667	1833.3333	3000	-0.0465	-0.0510	-0.0975
55.5	1150.0000	1850.0000	3000	-0.0461	-0.0508	-0.0969
56	1133.3333	1866.6667	3000	-0.0457	-0.0506	-0.0962
56.5	1116.6667	1883.3333	3000	-0.0452	-0.0503	-0.0956
57	1100.0000	1900.0000	3000	-0.0448	-0.0500	-0.0949
57.5	1083.3333	1916.6667	3000	-0.0444	-0.0498	-0.0941
58	1066.6667	1933.3333	3000	-0.0439	-0.0494	-0.0934
58.5	1050.0000	1950.0000	3000	-0.0435	-0.0491	-0.0926
59	1033.3333	1966.6667	3000	-0.0430	-0.0488	-0.0917
59.5	1016.6667	1983.3333	3000	-0.0425	-0.0484	-0.0909
60	1000.0000	2000.0000	3000	-0.0420	-0.0480	-0.0900
60.5	983.3333	1966.6667	2950	-0.0415	-0.0476	-0.0891
61	966.6667	1933.3333	2900	-0.0410	-0.0472	-0.0881
61.5	950.0000	1900.0000	2850	-0.0405	-0.0467	-0.0872
62	933.3333	1866.6667	2800	-0.0399	-0.0462	-0.0862
62.5	916.6667	1833.3333	2750	-0.0394	-0.0458	-0.0851
63	900.0000	1800.0000	2700	-0.0388	-0.0453	-0.0841
63.5	883.3333	1766.6667	2650	-0.0383	-0.0447	-0.0830
64	866.6667	1733.3333	2600	-0.0377	-0.0442	-0.0819
64.5	850.0000	1700.0000	2550	-0.0371	-0.0436	-0.0807
65	833.3333	1666.6667	2500	-0.0365	-0.0431	-0.0796
65.5	816.6667	1633.3333	2450	-0.0359	-0.0425	-0.0784
66	800.0000	1600.0000	2400	-0.0353	-0.0419	-0.0772
66.5	783.3333	1566.6667	2350	-0.0347	-0.0412	-0.0759
67	766.6667	1533.3333	2300	-0.0341	-0.0406	-0.0747
67.5	750.0000	1500.0000	2250	-0.0335	-0.0399	-0.0734
68	733.3333	1466.6667	2200	-0.0328	-0.0393	-0.0721
68.5	716.6667	1433.3333	2150	-0.0322	-0.0386	-0.0708
69	700.0000	1400.0000	2100	-0.0315	-0.0379	-0.0694
69.5	683.3333	1366.6667	2050	-0.0309	-0.0372	-0.0681
70	666.6667	1333.3333	2000	-0.0302	-0.0364	-0.0667
70.5	650.0000	1300.0000	1950	-0.0296	-0.0357	-0.0653
71	633.3333	1266.6667	1900	-0.0289	-0.0350	-0.0638
71.5	616.6667	1233.3333	1850	-0.0282	-0.0342	-0.0624
72	600.0000	1200.0000	1800	-0.0275	-0.0334	-0.0609
72.5	583.3333	1166.6667	1750	-0.0268	-0.0326	-0.0594
73	566.6667	1133.3333	1700	-0.0261	-0.0318	-0.0579
73.5	550.0000	1100.0000	1650	-0.0254	-0.0310	-0.0564
74	533.3333	1066.6667	1600	-0.0247	-0.0302	-0.0549
74.5	516.6667	1033.3333	1550	-0.0240	-0.0293	-0.0533
75	500.0000	1000.0000	1500	-0.0233	-0.0285	-0.0518
75.5	483.3333	966.6667	1450	-0.0225	-0.0276	-0.0502
76	466.6667	933.3333	1400	-0.0218	-0.0268	-0.0486
76.5	450.0000	900.0000	1350	-0.0211	-0.0259	-0.0470
77	433.3333	866.6667	1300	-0.0203	-0.0250	-0.0453
77.5	416.6667	833.3333	1250	-0.0196	-0.0241	-0.0437
78	400.0000	800.0000	1200	-0.0188	-0.0232	-0.0420
78.5	383.3333	766.6667	1150	-0.0181	-0.0223	-0.0404
79	366.6667	733.3333	1100	-0.0173	-0.0214	-0.0387
79.5	350.0000	700.0000	1050	-0.0165	-0.0205	-0.0370
80	333.3333	666.6667	1000	-0.0158	-0.0196	-0.0353
80.5	316.6667	633.3333	950	-0.0150	-0.0186	-0.0336
81	300.0000	600.0000	900	-0.0142	-0.0177	-0.0319
81.5	283.3333	566.6667	850	-0.0135	-0.0167	-0.0302
82	266.6667	533.3333	800	-0.0127	-0.0158	-0.0285
82.5	250.0000	500.0000	750	-0.0119	-0.0148	-0.0267
83	233.3333	466.6667	700	-0.0111	-0.0138	-0.0250
83.5	216.6667	433.3333	650	-0.0103	-0.0129	-0.0232
84	200.0000	400.0000	600	-0.0096	-0.0119	-0.0215
84.5	183.3333	366.6667	550	-0.0088	-0.0109	-0.0197
85	166.6667	333.3333	500	-0.0080	-0.0099	-0.0179
85.5	150.0000	300.0000	450	-0.0072	-0.0090	-0.0161
86	133.3333	266.6667	400	-0.0064	-0.0080	-0.0144
86.5	116.6667	233.3333	350	-0.0056	-0.0070	-0.0126
87	100.0000	200.0000	300	-0.0048	-0.0060	-0.0108
87.5	83.3333	166.6667	250	-0.0040	-0.0050	-0.0090
88	66.6667	133.3333	200	-0.0032	-0.0040	-0.0072
88.5	50.0000	100.0000	150	-0.0024	-0.0030	-0.0054
89	33.3333	66.6667	100	-0.0016	-0.0020	-0.0036
89.5	16.6667	33.3333	50	-0.0008	-0.0010	-0.0018
90	0.0000	0.0000	0	0.0000	0.0000	0.0000

Table 3a: Calculated theoretical moment, displacement, and bending stress along the beam length

x (in)	LC1 bending stress (psi)	LC2 bending stress (psi)	LC3 bending stress (psi)
0	0	0	0
0.5	20	10	30
1	40	20	60
1.5	60	30	90
2	80	40	120
2.5	100	50	150
3	120	60	180
3.5	140	70	210
4	160	80	240
4.5	180	90	270
5	200	100	300
5.5	220	110	330
6	240	120	360
6.5	260	130	390
7	280	140	420
7.5	300	150	450
8	320	160	480
8.5	340	170	510
9	360	180	540
9.5	380	190	570
10	400	200	600
10.5	420	210	630
11	440	220	660
11.5	460	230	690
12	480	240	720
12.5	500	250	750
13	520	260	780
13.5	540	270	810
14	560	280	840
14.5	580	290	870
15	600	300	900
15.5	620	310	930
16	640	320	960
16.5	660	330	990
17	680	340	1020
17.5	700	350	1050
18	720	360	1080
18.5	740	370	1110
19	760	380	1140
19.5	780	390	1170
20	800	400	1200
20.5	820	410	1230
21	840	420	1260
21.5	860	430	1290
22	880	440	1320
22.5	900	450	1350
23	920	460	1380
23.5	940	470	1410
24	960	480	1440
24.5	980	490	1470
25	1000	500	1500
25.5	1020	510	1530
26	1040	520	1560
26.5	1060	530	1590
27	1080	540	1620
27.5	1100	550	1650
28	1120	560	1680
28.5	1140	570	1710
29	1160	580	1740
29.5	1180	590	1770
30	1200	600	1800
30.5	1190	610	1800
31	1180	620	1800
31.5	1170	630	1800
32	1160	640	1800
32.5	1150	650	1800
33	1140	660	1800
33.5	1130	670	1800
34	1120	680	1800
34.5	1110	690	1800
35	1100	700	1800
35.5	1090	710	1800
36	1080	720	1800
36.5	1070	730	1800
37	1060	740	1800
37.5	1050	750	1800
38	1040	760	1800
38.5	1030	770	1800
39	1020	780	1800
39.5	1010	790	1800
40	1000	800	1800
40.5	990	810	1800
41	980	820	1800
41.5	970	830	1800
42	960	840	1800
42.5	950	850	1800
43	940	860	1800
43.5	930	870	1800
44	920	880	1800
44.5	910	890	1800
45	900	900	1800
45.5	890	910	1800
46	880	920	1800
46.5	870	930	1800
47	860	940	1800
47.5	850	950	1800
48	840	960	1800
48.5	830	970	1800
49	820	980	1800
49.5	810	990	1800
50	800	1000	1800
50.5	790	1010	1800
51	780	1020	1800
51.5	770	1030	1800
52	760	1040	1800
52.5	750	1050	1800
53	740	1060	1800
53.5	730	1070	1800
54	720	1080	1800
54.5	710	1090	1800
55	700	1100	1800
55.5	690	1110	1800
56	680	1120	1800
56.5	670	1130	1800
57	660	1140	1800
57.5	650	1150	1800
58	640	1160	1800
58.5	630	1170	1800
59	620	1180	1800
59.5	610	1190	1800
60	600	1200	1800
60.5	590	1180	1770
61	580	1160	1740
61.5	570	1140	1710
62	560	1120	1680
62.5	550	1100	1650
63	540	1080	1620
63.5	530	1060	1590
64	520	1040	1560
64.5	510	1020	1530
65	500	1000	1500
65.5	490	980	1470
66	480	960	1440
66.5	470	940	1410
67	460	920	1380
67.5	450	900	1350
68	440	880	1320
68.5	430	860	1290
69	420	840	1260
69.5	410	820	1230
70	400	800	1200
70.5	390	780	1170
71	380	760	1140
71.5	370	740	1110
72	360	720	1080
72.5	350	700	1050
73	340	680	1020
73.5	330	660	990
74	320	640	960
74.5	310	620	930
75	300	600	900
75.5	290	580	870
76	280	560	840
76.5	270	540	810
77	260	520	780
77.5	250	500	750
78	240	480	720
78.5	230	460	690
79	220	440	660
79.5	210	420	630
80	200	400	600
80.5	190	380	570
81	180	360	540
81.5	170	340	510
82	160	320	480
82.5	150	300	450
83	140	280	420
83.5	130	260	390
84	120	240	360
84.5	110	220	330
85	100	200	300
85.5	90	180	270
86	80	160	240
86.5	70	140	210
87	60	120	180
87.5	50	100	150
88	40	80	120
88.5	30	60	90
89	20	40	60
89.5	10	20	30
90	0	0	0

Table 3b: Continued theoretical calculations

X (in)	100lb at P1 (%)	100lb at P2 (%)	100lb at P1/P2 (%)
5	10.61	6.62	8.84
25	5.68	9.51	6.81
45	4.35	6.28	4.83
65	4.03	6.84	5.55
85	5.92	4.47	2.33

Table 4: Error analysis

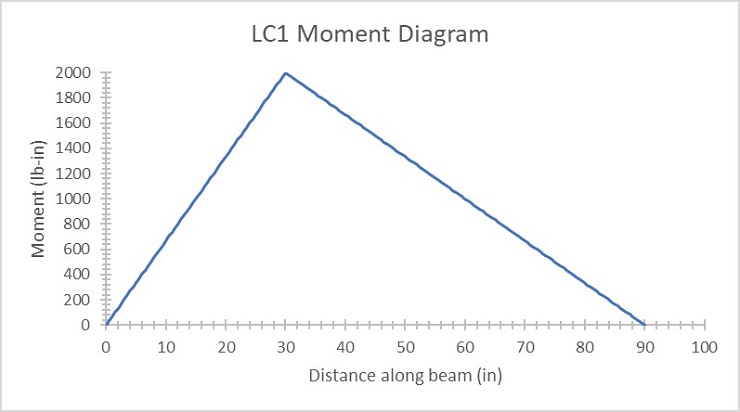
Figure 1: Load case 1 moment diagram

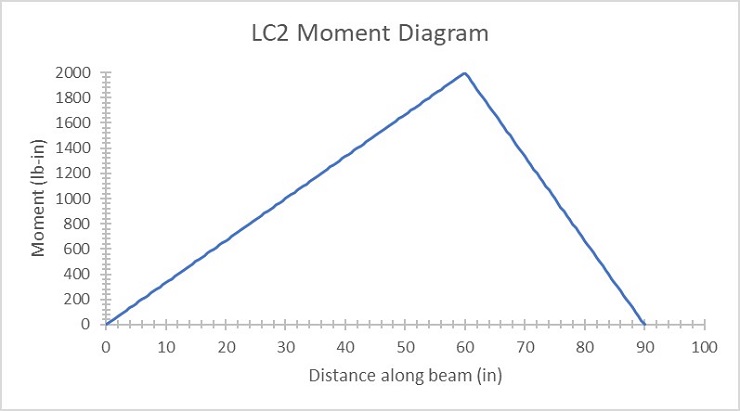
Figure 2: Load case 2 moment diagram

Figure 3: Load case 3 moment diagram

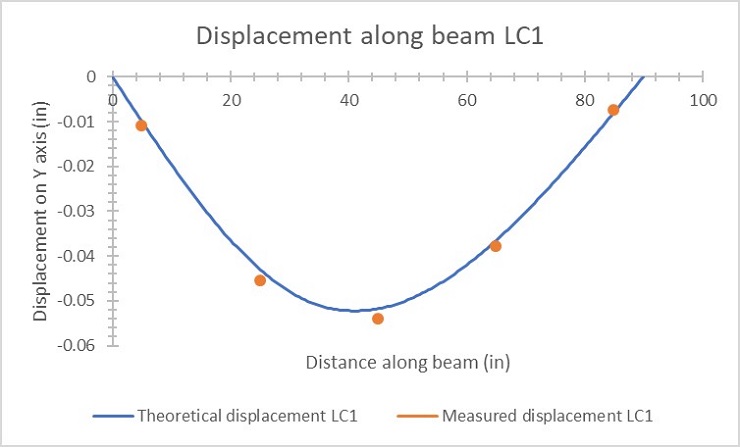
Figure 4: Load case 1 displacement

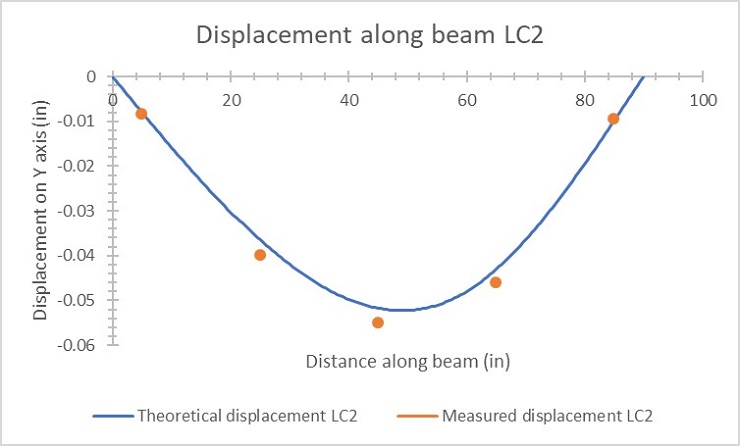
Figure 5: Load case 2 displacement

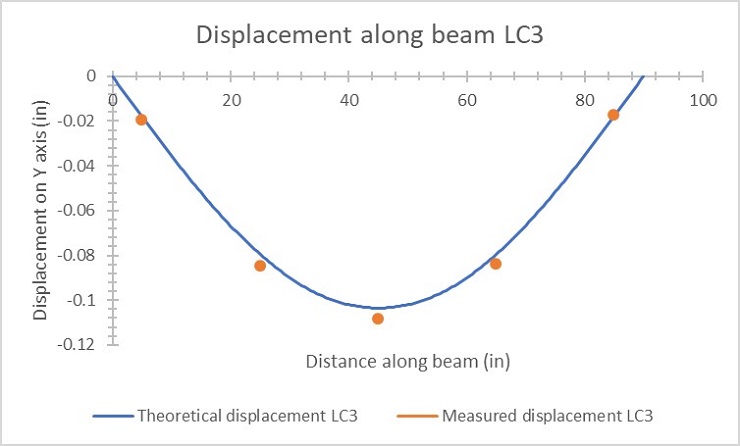
Figure 6: Load case 3 displacement

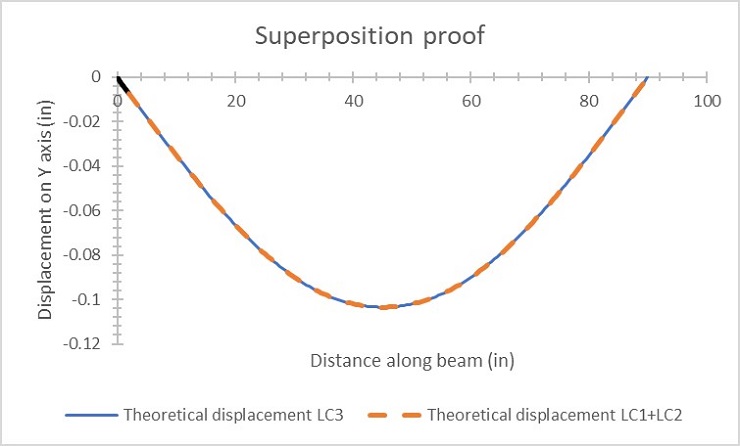
Figure 7: Super position proof

Discussion:

Our theoretical values very closely matched the data gathered during the experiment and were within the margin of error that comes from the theoretical calculations not factoring in the deformation caused by shear which was included in the measurements by the dial indicators. The differences between the calculated theoretical displacement was only off by a few thousandths of an inch at any given point where a dial indicator was located, which is to be expected. The displacement of the beam in the vertical direction is mostly caused by flexural deformation, but shear deformation factors into that as well, albeit a very small amount. This is why the displacement measured by the dial indicator is slightly larger than the values that were calculated. This accounts for most of the error found between the two values. Aside from this error, the experimental and theoretical data line up very closely and follow nearly identical trends when graphed against each other, which can be seen in figures 4-6. Another source of error is the high likelihood that the dial indicators were not perfectly perpendicular to the beam when placed. Once zeroed on the beam, they measure the distance changed on only one axis which would be the vertical axis in this case. If they were not perfectly perpendicular, they would be measuring the hypotenuse of a triangle rather than the vertical distance traveled. All of the length measurements and positioning of the loads as well as dial indicators were placed using a tape measure, which adds yet another source of error. During the experiment, point load 2 shifted slightly between runs two and three as the weights were loaded and unloaded. The weights used not all being exactly 20 pounds may also add more error to the calculations.

Comparing load cases one and two, they display essentially equal and opposite deformation reactions in the beam. To prove the theory of superposition, the deformation reactions from case one and two were added together and should be equal to case three since case three was the loads from cases one and two applied at the same time. This is proven to be true as evidenced by the graph in Figure 7 where the sum of cases one and two overlap the line of case three. The moment diagrams for all three cases are also related and the visual representations make sense since the internal moment should peak where the single loads are applied and when both loads are applied in case three, the moment between the loads should stay constant like it does.

Resources

24 x 7 Availability.
Trained and Certified Experts.
Deadline Guaranteed.
Plagiarism Free.
Privacy Guaranteed.
Free download.
Online help for all project.
Homework Help Services

Not the Exact Question you were looking for ? Post your question for assignment help and get instant help on your homework and assignment questions from our experts