Discrete Mathematics Assignment Help

Hire High Quality Discrete Mathematics Assignment Help Experts To Get Top Grades

Discrete Mathematics is the branch of Mathematics dealing with objects that can assume only distinct, separated values. The term {"Discrete Mathematics"} is therefore used in contrast with {"Continuous Mathematics,"} which is the branch of Mathematics dealing with objects that can vary smoothly (and which includes, for example, calculus). Whereas Discrete objects can often be characterized by Integers, continuous objects require Real Numbers.

Avail Benefits of Our Discrete Mathematics Assignment Help and Writing Services

The study of how discrete objects combine with one another and the probabilities of various outcomes is known as combinatorics. Other fields of Mathematics that are considered to be part of Discrete Mathematics include Graph Theory and the theory of computation. Topics in Number Theory such as congruences and recurrence relations are also considered part of Discrete Mathematics.

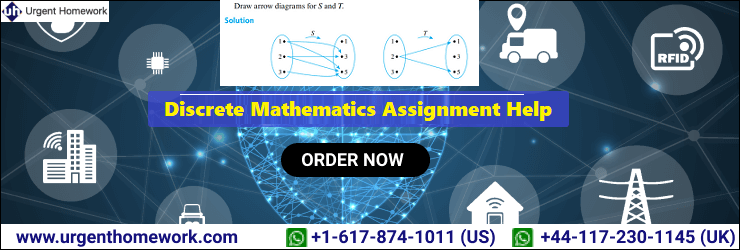
{" "} ---reference:{" "} DiscreteMathematics

Urgent Homework Help {" "} provides best{" "} Online Help ,{" "} Homework Help {" "} for Student who experiencing difficulties in Discrete Mathematics and its Application; Our{" "} Maths {" "} Experts can analyze your problem help you to understand and solve it. They are well skills people having years of experience in the area, Urgenthomework provides you the best of the specialized professionals to fulfill your all academic requirements.

Sample of Discrete Maths Assignment Help Solved by the Experts

                    Q1. In this question, write down your answer, no need for
                    any justification. You can leave your answer in terms of
                    factorials , combination symbols, permutation symbols, etc.
                    Please clearly box your answers in your submission to
                    Gradescope.
                  

• How many nonisomorphic (free) trees are there with 4 vertices?
• How many solutions are there to x ₁+x ₂+x ₃+x ₄ = 20 where x ₄ ≥ 10 and each{" "} x_i is a natural number (0 counts as a natural number for us).
• Let X = {1,2,3 ,4,5}. How many relations are there on X with the property that for all x ∈ X, x is not related to itself?
• Give the equivalence relation on{" "} } a,b,c,d,e} whose equivalence classes give the partition P ={" "} { {a,b},{c,d},{e} .
• You are dividing 112 apples among 5 boxes. What is the smallest number of apples that could appear in the box with the most apples?

                    2. Let X be a set with n elements. Be sure to justify all
                    your answers.
                  

• (3 points) How many reflexive relations are there on{" "} X?
• (4 points) How many antisymmetric relations are there on{" "} X?
• (3 points) How many reflexive or antisymmetric relations are there on X?
• (4 points) Let{" "} B_n denote the number of partitions of a set with n elements and set B ₀ = 1.

3. 3. Show that Bn satisfies the recurrence Bn .

(3 points) Show that for all n ≥ 1, 2 ^{n− 1} ≤ B_n .

(3 points) Show that for n ≥ 1,{" "} B_n ≤{" "} 2 ^{n 2}. Hint: Remember that for a nonempty set there is a bijection between partitions on that set and equivalence relations on that set.

• (a) (5 points) Give a formula for the number of subgraphs of{" "} K_n that have exactly n vertices (and prove that it is correct)
  • (5 points) Give a formula for the number of subgraphs of{" "} K_n (and prove that it is correct).
• (a) (2 points) Show that every cycle in the n -cube is of length 4 or longer.
  • (2 points) How many edges does the n-cube have?
  • (2 points) If the n-cube was planar, how many faces would it have? (Your answer will depend on{" "} n).
  • (4 points) Show that the 4-cube (and hence every{" "} n-cube with n ≥ 4) is not planar.

• (a) (5 points) Suppose that G is a simple connected graph with finitely many vertices, and suppose that e is an edge in G such that removing e from G results in a disconnected graph. Show that e is in every spanning tree of G.
  • (5 points) Suppose that G is a simple connected weighted graph with finitely many vertices and that if distinct edges e and{" "} e⁰ in G have the same weight, then removing either e or{" "} e⁰ from G results in a disconnected graph.

Show that G has a unique minimal spanning tree.

• A perfect binary tree of height h is a binary tree of height h with 2 ^h terminal vertices.
  • (5 points) Show that if T is a perfect binary tree of height h then the left and right subtrees of the root are each perfect binary trees of height h −
  • (5 points) Show that if T ₁ and T ₂ are each perfect binary trees of height{" "} h then T ₁ and T ₂ are isomorphic as binary trees.
• A 3-ary tree is a rooted tree where each parent has at most three children, and each child is labeled with 1, 2, or 3 (and siblings all have different labels). A full 3-ary tree is a 3-ary tree where each parent has exactly 3 children.

Two 3-ary trees are isomorphic as 3-ary trees if they are isomorphic as rooted trees and the isomorphism preserves the labels of the children.

• (5 points) Show that there is a bijection between the set of nonisomorphic (as 3-ary trees) 3-ary trees with{" "} n vertices and the set of nonisomorphic (as 3-ary trees) full 3-ary trees with 2n + 1 terminal vertices.
• (5 points) Let{" "} t_n be the number of nonisomorphic (as 3-ary trees) 3-ary trees with n vertices, and by convention set{" "} t ₀ = 1.

Show that t n i t it jt n−1−(i+j).